View that there are important paraconsistent logical theories; that is theories that do not allow (as classical logic does: see relevance logics) that a contradiction has every proposition among its logical consequences.
A system which contains contradictory proposition is inconsistent. But if it does not also contain every proposition (as it would for classical logic) it avoids being trivial. It is claimed that various scientific and mathematical theories are in fact of this nature, and so can be logically analyzed by paraconsistent logics, and also shown to be respectable as they stand (that is, we need not assume that the inconsistencies in them are merely aberrations that must be removed before they can be properly studied).
Also see: dialetheism
Source:
G Priest and R Routley and J Norman, eds, Paraconsistent Logic (1985)
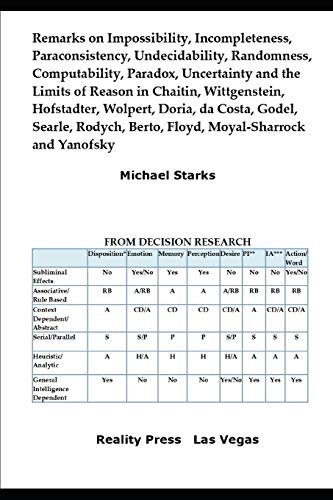
Table of Contents
- 1 Videos
- 2 Related Products
- 2.1 Paraconsistency: Logic and Applications (Logic, Epistemology, and the Unity of Science Book 26)
- 2.2 Graham Priest on Dialetheism and Paraconsistency (Outstanding Contributions to Logic)
- 2.3 Paraconsistency: The Logical Way to the Inconsistent
- 2.4 Paraconsistent Intelligent-Based Systems: New Trends in the Applications of Paraconsistency (Intelligent Systems Reference Library)
- 2.5 Constructive Negations and Paraconsistency (Trends in Logic Book 26)
- 2.6 Remarks on Impossibility, Incompleteness, Paraconsistency, Undecidability, and Randomness: Chaitin, Wittgenstein, Hofstadter, Wolpert, Doria, da Costa, Godel, Searle, Rodych, Berto, and Floyd
- 2.7 Remarks on Impossibility, Incompleteness, Paraconsistency, Undecidability, Randomness, Computability, Paradox, and Uncertainty: in Chaitin, ... Berto, Floyd, Moyal-Sharrock and Yanofsky
- 2.8 Paradox and Paraconsistency: Conflict Resolution in the Abstract Sciences
- 2.9 Handbook of Paraconsistency (Studies in Logic)
- 2.10 Paraconsistency: The Logical Way to the Inconsistent
Videos
Paraconsistency: Logic and Applications (Logic, Epistemology, and the Unity of Science Book 26)
- Amazon Kindle Edition
- English (Publication Language)
- 392 Pages - 07/26/2012 (Publication Date) - Springer (Publisher)
Graham Priest on Dialetheism and Paraconsistency (Outstanding Contributions to Logic)
- Hardcover Book
- English (Publication Language)
- 704 Pages - 01/02/2020 (Publication Date) - Springer (Publisher)
Paraconsistency: The Logical Way to the Inconsistent
- Amazon Kindle Edition
- Coniglio, Marcelo E. (Author)
- English (Publication Language)
- 04/17/2007 (Publication Date) - Dekker (Publisher)
Paraconsistent Intelligent-Based Systems: New Trends in the Applications of Paraconsistency (Intelligent Systems Reference Library)
- Hardcover Book
- English (Publication Language)
- 306 Pages - 06/23/2015 (Publication Date) - Springer (Publisher)
Constructive Negations and Paraconsistency (Trends in Logic Book 26)
- Amazon Kindle Edition
- Odintsov, Sergei (Author)
- English (Publication Language)
- 242 Pages - 03/19/2008 (Publication Date) - Springer (Publisher)
Remarks on Impossibility, Incompleteness, Paraconsistency, Undecidability, and Randomness: Chaitin, Wittgenstein, Hofstadter, Wolpert, Doria, da Costa, Godel, Searle, Rodych, Berto, and Floyd
- Amazon Kindle Edition
- starks, michael (Author)
- English (Publication Language)
- 138 Pages - 08/13/2019 (Publication Date) - Reality Press (Publisher)
Remarks on Impossibility, Incompleteness, Paraconsistency, Undecidability, Randomness, Computability, Paradox, and Uncertainty: in Chaitin, ... Berto, Floyd, Moyal-Sharrock and Yanofsky
- starks, michael (Author)
- English (Publication Language)
- 77 Pages - 08/14/2019 (Publication Date) - Independently published (Publisher)
Paradox and Paraconsistency: Conflict Resolution in the Abstract Sciences
- Amazon Kindle Edition
- Woods, John (Author)
- English (Publication Language)
- 382 Pages - 09/19/2002 (Publication Date) - Cambridge University Press (Publisher)
Handbook of Paraconsistency (Studies in Logic)
- English (Publication Language)
- 528 Pages - 11/01/2007 (Publication Date) - College Publications (Publisher)
Paraconsistency: The Logical Way to the Inconsistent
- Hardcover Book
- Carnielli, Walter Alexandr (Author)
- English (Publication Language)
- 576 Pages - 10/02/2019 (Publication Date) - CRC Press (Publisher)
Last update 2020-06-17. Price and product availability may change.